| << problem 85 - Counting rectangles |

|
Prime power triples - problem 87 >> |
Problem 86: Cuboid route
(see projecteuler.net/problem=86)
A spider, S, sits in one corner of a cuboid room, measuring 6 by 5 by 3, and a fly, F, sits in the opposite corner.
By travelling on the surfaces of the room the shortest "straight line" distance from S to F is 10 and the path is shown on the diagram.
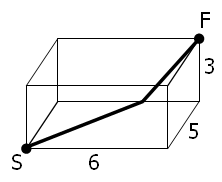
However, there are up to three "shortest" path candidates for any given cuboid and the shortest route doesn't always have integer length.
It can be shown that there are exactly 2060 distinct cuboids, ignoring rotations, with integer dimensions,
up to a maximum size of M by M by M, for which the shortest route has integer length when M = 100.
This is the least value of M for which the number of solutions first exceeds two thousand; the number of solutions when M = 99 is 1975.
Find the least value of M such that the number of solutions first exceeds one million.
My Algorithm
Let's assume a is the longest side of the cuboid, b and c are shorter: a >= b >= c
If I unfold the cuboid, then the spider walks d = sqrt{a^2 + (b+c)^2}
All I have to do is counting all integer solutions of this equation ... which took me some time.
In case I find such a valid pair (a, b+c) where the shortest path is an integer then combinations
will return the number of different cuboids (a, b, c) that can be constructed.
1. if a is longer than b+c:
any combination (b,c) where b >= c and b+c = b_c produces a valid cuboid (a,b,c) and there are dfrac{b+c}{2} such combinations
e.g. b_c = 7 → (b,c) = {(6,1), (5,2), (4,3)} → \lfloor 7/2 \rfloor = 3 elements
e.g. b_c = 10 → (b,c) = {(9,1), (8,2), (7,3), (6,4), (5,5)} → \lfloor 10/2 \rfloor = 5 elements
2. else a < b+c. Keep in mind that a is still the longest side, so some combinations of (b,c) must be rejected:
a >= b >= c means that a >= b >= dfrac{b+c}{2} because b >= c
e.g. a = 6 and b_c = 8 → (a,b,c) = {(6,6,2), (6,5,3), (6,4,4)} → 3 elements, rejected (6,7,1) because a \ngeq b
I wrote two functions:
countSinglereturns the number of paths for a given side lengthacountAllreturns the number of paths for all cuboids whereadoesn't exceed the parameterlimit
countSingle is much slower for large input(but still finds the solution to the original problem in less than 0.05 seconds).
countSingle follows a pseudo-brute-force approach and analyzes all potential b_c = b + c whether they form a valid triangle.It was the first code I had for this problem but couldn't solve the much larger Hackerrank input space.
countAll evaluates Euclid's formula (see en.wikipedia.org/wiki/Pythagorean_triple) to find all basic triangles and all of their multiples.
Interactive test
You can submit your own input to my program and it will be instantly processed at my server:
This live test is based on the Hackerrank problem.
This is equivalent toecho "1 100" | ./86
Output:
(this interactive test is still under development, computations will be aborted after one second)
My code
… was written in C++11 and can be compiled with G++, Clang++, Visual C++. You can download it, too. Or just jump to my GitHub repository.
The code contains #ifdefs to switch between the original problem and the Hackerrank version.
Enable #ifdef ORIGINAL to produce the result for the original problem (default setting for most problems).
#include <cmath> #include <vector> #include <iostream> // oeis.org/A143714
// how many combinations a,b,c exist for b_c = b + c
unsigned int combinations(unsigned int a, unsigned int b_c) { // the longest side must be a => if 2a < b + c then either b or c (or both !) would be longer than a if (2*a < b_c) return 0; // if a is longer than b+c: // any combination (b,c) where b >= c and b+c = b_c produces a valid cuboid (a,b,c), // there are (b+c) / 2 such combinations // e.g. b_c = 7 => (b,c) = {(6,1), (5,2), (4,3)} => 7/2 = 3 elements // e.g. b_c = 10 => (b,c) = {(9,1), (8,2), (7,3), (6,4), (5,5)} => 10/2 = 5 elements if (a >= b_c) return b_c / 2; // now a < b+c: // a is still the longest side, but some combinations of (b,c) must be rejected: // a >= b >= c means that a >= b >= (b+c)/2 because b >= c // e.g. a = 6 and b_c = 8 => (a,b,c) = {(6,6,2), (6,5,3), (6,4,4)}, rejected (6,7,1) return a - (b_c - 1) / 2; // b+c minus one because b = c is not rejected } // count how many paths exists with length a (mediocre speed)
unsigned long long countSingle(unsigned int a) { unsigned long long sum = 0; // we encounter the same sum b+c many times (for different b and c) // lets try it the other way around: // step through all sums b+c and figure out the possible combinations of b and c // since a >= b and a >= c we also know 2*a >= b+c // I'll call b+c from now on "b_c" for (unsigned int b_c = 1; b_c <= 2 * a; b_c++) { // triangle with right angle ? auto diagonalSquared = a * a + b_c * b_c; unsigned int root = sqrt(diagonalSquared); // diagionalSquared needs to be a perfect square if (root * root == diagonalSquared) sum += combinations(a, b_c); } return sum; } // greatest common divisor
unsigned int gcd(unsigned int x, unsigned int y) { while (x != 0) { auto temp = x; x = y % x; y = temp; } return y; } // count combinations per path length
std::vector<unsigned long long> countAll(unsigned int limit) { // result std::vector<unsigned long long> solutions(limit + 1, 0); // find basic Pythagorean triples for (unsigned int m = 1; m <= sqrt(2*limit); m++) for (unsigned int n = 1; n < m; n++) { if (m % 2 == n % 2) // if the inner loop is (n = (m%2)+1; n < m; n += 2) continue; // then this check could be removed if (gcd(m, n) != 1) continue; // two sides auto x = m*m - n*n; auto y = 2*m*n; //auto z = m*m + n*n; // ... and now scan all multiples for (unsigned int k = 1; k*x <= limit; k++) solutions[k*x] += combinations(k*x, k*y); for (unsigned int k = 1; k*y <= limit; k++) solutions[k*y] += combinations(k*y, k*x); } return solutions; } int main() { //#define ORIGINAL
#ifdef ORIGINAL unsigned int sum = 0; unsigned int a = 0; // until exceeding one million while (sum <= 1000000) sum += countSingle(++a); // print result std::cout << a << std::endl; #else // count combinations per path length auto solutions = countAll(1000000); // compute sum of all path lengths std::vector<unsigned long long> total; unsigned long long sum = 0; for (auto i : solutions) { sum += i; total.push_back(sum); } unsigned int tests; std::cin >> tests; while (tests--) { unsigned int maxA; std::cin >> maxA; // simple lookup std::cout << total[maxA] << std::endl; } #endif return 0; }
This solution contains 20 empty lines, 33 comments and 6 preprocessor commands.
Benchmark
The correct solution to the original Project Euler problem was found in 0.03 seconds on an Intel® Core™ i7-2600K CPU @ 3.40GHz.
(compiled for x86_64 / Linux, GCC flags: -O3 -march=native -fno-exceptions -fno-rtti -std=gnu++11 -DORIGINAL)
See here for a comparison of all solutions.
Note: interactive tests run on a weaker (=slower) computer. Some interactive tests are compiled without -DORIGINAL.
Changelog
March 15, 2017 submitted solution
May 9, 2017 added comments
Hackerrank
see https://www.hackerrank.com/contests/projecteuler/challenges/euler086
My code solves 10 out of 10 test cases (score: 100%)
Difficulty
Project Euler ranks this problem at 35% (out of 100%).
Hackerrank describes this problem as hard.
Note:
Hackerrank has strict execution time limits (typically 2 seconds for C++ code) and often a much wider input range than the original problem.
In my opinion, Hackerrank's modified problems are usually a lot harder to solve. As a rule thumb: brute-force is rarely an option.
Links
projecteuler.net/thread=86 - the best forum on the subject (note: you have to submit the correct solution first)
Code in various languages:
C# www.mathblog.dk/project-euler-86-shortest-path-cuboid/ (written by Kristian Edlund)
C# github.com/HaochenLiu/My-Project-Euler/blob/master/086.cs (written by Haochen Liu)
Python github.com/nayuki/Project-Euler-solutions/blob/master/python/p086.py (written by Nayuki)
Python github.com/smacke/project-euler/blob/master/python/86.py (written by Stephen Macke)
Python github.com/steve98654/ProjectEuler/blob/master/086.py
C++ github.com/Meng-Gen/ProjectEuler/blob/master/86.cc (written by Meng-Gen Tsai)
C++ github.com/roosephu/project-euler/blob/master/86.cpp (written by Yuping Luo)
C++ github.com/steve98654/ProjectEuler/blob/master/086.cpp
Java github.com/dcrousso/ProjectEuler/blob/master/PE086.java (written by Devin Rousso)
Java github.com/nayuki/Project-Euler-solutions/blob/master/java/p086.java (written by Nayuki)
Java github.com/thrap/project-euler/blob/master/src/Java/Problem86.java (written by Magnus Solheim Thrap)
Go github.com/frrad/project-euler/blob/master/golang/Problem086.go (written by Frederick Robinson)
Mathematica github.com/steve98654/ProjectEuler/blob/master/086.nb
Scala github.com/samskivert/euler-scala/blob/master/Euler086.scala (written by Michael Bayne)
Perl github.com/gustafe/projecteuler/blob/master/086-Cuboid-route.pl (written by Gustaf Erikson)
Perl github.com/shlomif/project-euler/blob/master/project-euler/86/euler-86.pl (written by Shlomi Fish)
Rust github.com/gifnksm/ProjectEulerRust/blob/master/src/bin/p086.rs
Those links are just an unordered selection of source code I found with a semi-automatic search script on Google/Bing/GitHub/whatever.
You will probably stumble upon better solutions when searching on your own.
Maybe not all linked resources produce the correct result and/or exceed time/memory limits.
Heatmap
Please click on a problem's number to open my solution to that problem:
| green | solutions solve the original Project Euler problem and have a perfect score of 100% at Hackerrank, too | |
| yellow | solutions score less than 100% at Hackerrank (but still solve the original problem easily) | |
| gray | problems are already solved but I haven't published my solution yet | |
| blue | solutions are relevant for Project Euler only: there wasn't a Hackerrank version of it (at the time I solved it) or it differed too much | |
| orange | problems are solved but exceed the time limit of one minute or the memory limit of 256 MByte | |
| red | problems are not solved yet but I wrote a simulation to approximate the result or verified at least the given example - usually I sketched a few ideas, too | |
| black | problems are solved but access to the solution is blocked for a few days until the next problem is published | |
| [new] | the flashing problem is the one I solved most recently |
I stopped working on Project Euler problems around the time they released 617.
| 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | 13 | 14 | 15 | 16 | 17 | 18 | 19 | 20 | 21 | 22 | 23 | 24 | 25 |
| 26 | 27 | 28 | 29 | 30 | 31 | 32 | 33 | 34 | 35 | 36 | 37 | 38 | 39 | 40 | 41 | 42 | 43 | 44 | 45 | 46 | 47 | 48 | 49 | 50 |
| 51 | 52 | 53 | 54 | 55 | 56 | 57 | 58 | 59 | 60 | 61 | 62 | 63 | 64 | 65 | 66 | 67 | 68 | 69 | 70 | 71 | 72 | 73 | 74 | 75 |
| 76 | 77 | 78 | 79 | 80 | 81 | 82 | 83 | 84 | 85 | 86 | 87 | 88 | 89 | 90 | 91 | 92 | 93 | 94 | 95 | 96 | 97 | 98 | 99 | 100 |
| 101 | 102 | 103 | 104 | 105 | 106 | 107 | 108 | 109 | 110 | 111 | 112 | 113 | 114 | 115 | 116 | 117 | 118 | 119 | 120 | 121 | 122 | 123 | 124 | 125 |
| 126 | 127 | 128 | 129 | 130 | 131 | 132 | 133 | 134 | 135 | 136 | 137 | 138 | 139 | 140 | 141 | 142 | 143 | 144 | 145 | 146 | 147 | 148 | 149 | 150 |
| 151 | 152 | 153 | 154 | 155 | 156 | 157 | 158 | 159 | 160 | 161 | 162 | 163 | 164 | 165 | 166 | 167 | 168 | 169 | 170 | 171 | 172 | 173 | 174 | 175 |
| 176 | 177 | 178 | 179 | 180 | 181 | 182 | 183 | 184 | 185 | 186 | 187 | 188 | 189 | 190 | 191 | 192 | 193 | 194 | 195 | 196 | 197 | 198 | 199 | 200 |
| 201 | 202 | 203 | 204 | 205 | 206 | 207 | 208 | 209 | 210 | 211 | 212 | 213 | 214 | 215 | 216 | 217 | 218 | 219 | 220 | 221 | 222 | 223 | 224 | 225 |
| 226 | 227 | 228 | 229 | 230 | 231 | 232 | 233 | 234 | 235 | 236 | 237 | 238 | 239 | 240 | 241 | 242 | 243 | 244 | 245 | 246 | 247 | 248 | 249 | 250 |
| 251 | 252 | 253 | 254 | 255 | 256 | 257 | 258 | 259 | 260 | 261 | 262 | 263 | 264 | 265 | 266 | 267 | 268 | 269 | 270 | 271 | 272 | 273 | 274 | 275 |
| 276 | 277 | 278 | 279 | 280 | 281 | 282 | 283 | 284 | 285 | 286 | 287 | 288 | 289 | 290 | 291 | 292 | 293 | 294 | 295 | 296 | 297 | 298 | 299 | 300 |
| 301 | 302 | 303 | 304 | 305 | 306 | 307 | 308 | 309 | 310 | 311 | 312 | 313 | 314 | 315 | 316 | 317 | 318 | 319 | 320 | 321 | 322 | 323 | 324 | 325 |
| 326 | 327 | 328 | 329 | 330 | 331 | 332 | 333 | 334 | 335 | 336 | 337 | 338 | 339 | 340 | 341 | 342 | 343 | 344 | 345 | 346 | 347 | 348 | 349 | 350 |
| 351 | 352 | 353 | 354 | 355 | 356 | 357 | 358 | 359 | 360 | 361 | 362 | 363 | 364 | 365 | 366 | 367 | 368 | 369 | 370 | 371 | 372 | 373 | 374 | 375 |
| 376 | 377 | 378 | 379 | 380 | 381 | 382 | 383 | 384 | 385 | 386 | 387 | 388 | 389 | 390 | 391 | 392 | 393 | 394 | 395 | 396 | 397 | 398 | 399 | 400 |
| 401 | 402 | 403 | 404 | 405 | 406 | 407 | 408 | 409 | 410 | 411 | 412 | 413 | 414 | 415 | 416 | 417 | 418 | 419 | 420 | 421 | 422 | 423 | 424 | 425 |
| 426 | 427 | 428 | 429 | 430 | 431 | 432 | 433 | 434 | 435 | 436 | 437 | 438 | 439 | 440 | 441 | 442 | 443 | 444 | 445 | 446 | 447 | 448 | 449 | 450 |
| 451 | 452 | 453 | 454 | 455 | 456 | 457 | 458 | 459 | 460 | 461 | 462 | 463 | 464 | 465 | 466 | 467 | 468 | 469 | 470 | 471 | 472 | 473 | 474 | 475 |
| 476 | 477 | 478 | 479 | 480 | 481 | 482 | 483 | 484 | 485 | 486 | 487 | 488 | 489 | 490 | 491 | 492 | 493 | 494 | 495 | 496 | 497 | 498 | 499 | 500 |
| 501 | 502 | 503 | 504 | 505 | 506 | 507 | 508 | 509 | 510 | 511 | 512 | 513 | 514 | 515 | 516 | 517 | 518 | 519 | 520 | 521 | 522 | 523 | 524 | 525 |
| 526 | 527 | 528 | 529 | 530 | 531 | 532 | 533 | 534 | 535 | 536 | 537 | 538 | 539 | 540 | 541 | 542 | 543 | 544 | 545 | 546 | 547 | 548 | 549 | 550 |
| 551 | 552 | 553 | 554 | 555 | 556 | 557 | 558 | 559 | 560 | 561 | 562 | 563 | 564 | 565 | 566 | 567 | 568 | 569 | 570 | 571 | 572 | 573 | 574 | 575 |
| 576 | 577 | 578 | 579 | 580 | 581 | 582 | 583 | 584 | 585 | 586 | 587 | 588 | 589 | 590 | 591 | 592 | 593 | 594 | 595 | 596 | 597 | 598 | 599 | 600 |
| 601 | 602 | 603 | 604 | 605 | 606 | 607 | 608 | 609 | 610 | 611 | 612 | 613 | 614 | 615 | 616 | 617 | 618 | 619 | 620 | 621 | 622 | 623 | 624 | 625 |
| 626 | 627 | 628 | 629 | 630 | 631 | 632 | 633 | 634 | 635 | 636 | 637 | 638 | 639 | 640 | 641 | 642 | 643 | 644 | 645 | 646 | 647 | 648 | 649 | 650 |
| 651 | 652 | 653 | 654 | 655 | 656 | 657 | 658 | 659 | 660 | 661 | 662 | 663 | 664 | 665 | 666 | 667 | 668 | 669 | 670 | 671 | 672 | 673 | 674 | 675 |
| 676 | 677 | 678 | 679 | 680 | 681 | 682 | 683 | 684 | 685 | 686 | 687 | 688 | 689 | 690 | 691 | 692 | 693 | 694 | 695 | 696 | 697 | 698 | 699 | 700 |
| 701 | 702 | 703 | 704 | 705 | 706 | 707 | 708 | 709 | 710 | 711 | 712 | 713 | 714 | 715 | 716 | 717 | 718 | 719 | 720 | 721 | 722 | 723 | 724 | 725 |
| 726 | 727 | 728 | 729 | 730 | 731 | 732 | 733 | 734 | 735 | 736 | 737 | 738 | 739 | 740 | 741 | 742 | 743 | 744 | 745 | 746 | 747 | 748 | 749 | 750 |
| 751 | 752 | 753 | 754 | 755 | 756 | 757 | 758 | 759 | 760 | 761 | 762 | 763 | 764 | 765 | 766 | 767 | 768 | 769 | 770 | 771 | 772 | 773 | 774 | 775 |
| 776 | 777 | 778 | 779 | 780 | 781 | 782 | 783 | 784 | 785 | 786 | 787 | 788 | 789 | 790 | 791 | 792 | 793 | 794 | 795 | 796 | 797 | 798 | 799 | 800 |
| 801 | 802 | 803 | 804 | 805 | 806 | 807 | 808 | 809 |
I scored 13526 points (out of 15700 possible points, top rank was 17 out of ≈60000 in August 2017) at Hackerrank's Project Euler+.
My username at Project Euler is stephanbrumme while it's stbrumme at Hackerrank.
Look at my progress and performance pages to get more details.
Copyright
I hope you enjoy my code and learn something - or give me feedback how I can improve my solutions.
All of my solutions can be used for any purpose and I am in no way liable for any damages caused.
You can even remove my name and claim it's yours. But then you shall burn in hell.
The problems and most of the problems' images were created by Project Euler.
Thanks for all their endless effort !!!
| << problem 85 - Counting rectangles |

|
Prime power triples - problem 87 >> |